The lack of pressure support from thermonuclear reactions in the star will allow the matter to be compressed very tightly by gravity. Consider the positive nuclei of the core material. These will be experiencing mutual repulsion. If the kinetic energy (ie temperature) of the nuclei is low enough they will form a stable lattice structure.
As has been seen earlier, the degeneracy of the electrons gives them a large average kinetic energy. This is of the order of 104eV, whereas the atomic binding energy of the nucleus (for helium) is 23 eV, giving rise to a delocalised electron cloud. The screening effect of the electrons reduces the charge felt by each nucleus to about 1/8 of that without any electrons.
The balance between the kinetic energy of the nuclei and the Coulomb repulsion will determine whether the nuclei will form a solid lattice, or exist in a liquid or gas phase. If l is the spacing between adjacent nuclei, Z the nuclear charge, e the charge on the electron, and k Boltzmann’s constant., then the following factors determine the structure of the lattice:
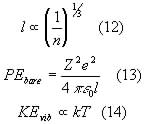
For high densities, as do exist in the situations that we are looking at, the PEbare dominates over the KEvib and a lattice of nuclei exists. The electrons however, are largely undeflected by the lattice, as they possess such large kinetic energies, and they move in virtually a straight line throughout the lattice.